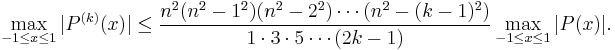Markov brothers' inequality

In mathematics, the Markov brothers' inequality is an inequality proved by Andrey Markov and Vladimir Markov. This inequality bounds the maximum of the derivatives of a polynomial on an interval in terms of the maximum of the polynomial.[1] For k = 1 it was proved by Andrey Markov,[2] and for k = 2,3,... by his brother Vladimir Markov.[3]

The statement

Let P be a polynomial of degree ≤ n. Then

\max_{-1 \leq x \leq 1} |P^{(k)}(x)| \leq \frac{n^2 (n^2 - 1^2) (n^2 - 2^2) \cdots (n^2 - (k-1)^2)}{1 \cdot 3 \cdot 5 \cdots (2k-1)} \max_{-1 \leq x \leq 1} |P(x)|.

Equality is attained for Chebyshev polynomials of the first kind.

Related inequalities

References

  1. ^ Achiezer, N.I. (1992). Theory of approximation. New York: Dover Publications, Inc.. 
  2. ^ Markov, A.A. (1890). "On a question by D. I. Mendeleev". Zap. Imp. Akad. Nauk SPb. 62: 1–24. 
  3. ^ Markov, V.A. (1892). О функциях, наименее уклоняющихся от нуля в данном промежутке (On Functions of Least Deviation from Zero in a Given Interval).  Appeared in German with a foreword by Sergei Bernstein as Markov, V.A. (1916). "Über Polynome, die in einem gegebenen Intervalle möglichst wenig von Null abweichen". Math. Ann. 77: 213–258.